7.12 Scat­ter­ing

The mo­tion of the wave pack­ets in sec­tion 7.11 ap­prox­i­mated that of clas­si­cal New­ton­ian par­ti­cles. How­ever, if the po­ten­tial starts vary­ing non­triv­ially over dis­tances short enough to be com­pa­ra­ble to a quan­tum wave length, much more in­ter­est­ing be­hav­ior re­sults, for which there is no clas­si­cal equiv­a­lent. This sec­tion gives a cou­ple of im­por­tant ex­am­ples.

7.12.1 Par­tial re­flec­tion

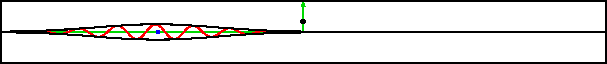
A clas­si­cal par­ti­cle en­ter­ing a re­gion of chang­ing po­ten­tial will keep go­ing as long as its to­tal en­ergy ex­ceeds the po­ten­tial en­ergy. Con­sider the po­ten­tial shown in green in fig­ure 7.19; it drops off to a lower level and then stays there. A clas­si­cal par­ti­cle would ac­cel­er­ate to a higher speed in the re­gion of drop off and main­tain that higher speed from there on.

Fig­ure 7.19: A par­tial re­flec­tion.

 

Move your mouse over the bot­tom fig­ure to see the an­i­ma­tion. Javascript must be en­abled on your browser. Give it a few sec­onds for the an­i­ma­tion to load, es­pe­cially on a phone line.
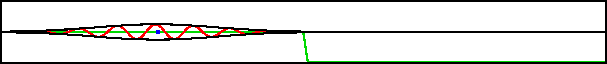
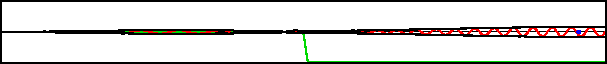
Im­proved res­o­lu­tion ver­sion (566 kB)

How­ever, the po­ten­tial in this ex­am­ple varies so rapidly on quan­tum scales that the clas­si­cal New­ton­ian pic­ture is com­pletely wrong. What ac­tu­ally hap­pens is that the wave packet splits into two, as shown in the bot­tom fig­ure. One part re­turns to where the packet came from, the other keeps on go­ing.

One hy­po­thet­i­cal ex­am­ple used in chap­ter 3.1 was that of send­ing a sin­gle par­ti­cle both to Venus and to Mars. As this ex­am­ple shows, a scat­ter­ing setup gives a very real way of send­ing a sin­gle par­ti­cle in two dif­fer­ent di­rec­tions at the same time.

Par­tial re­flec­tions are the norm for po­ten­tials that vary non­triv­ially on quan­tum scales, but this ex­am­ple adds a sec­ond twist. Clas­si­cally, a de­cel­er­at­ing force is needed to turn a par­ti­cle back, but here the force is every­where ac­cel­er­at­ing only! As an ac­tual phys­i­cal ex­am­ple of this weird be­hav­ior, neu­trons try­ing to en­ter nu­clei ex­pe­ri­ence at­trac­tive forces that come on so quickly that they may be re­pelled by them.

7.12.2 Tun­nel­ing

A clas­si­cal par­ti­cle will never be able to progress past a point at which the po­ten­tial en­ergy ex­ceeds its to­tal en­ergy. It will be turned back. How­ever, the quan­tum me­chan­i­cal truth is, if the re­gion in which the po­ten­tial en­ergy ex­ceeds the par­ti­cle's en­ergy is nar­row enough on a quan­tum scale, the par­ti­cle can go right through it. This ef­fect is called tun­nel­ing.

As an ex­am­ple, fig­ure 7.20 shows part of the wave packet of a par­ti­cle pass­ing right through a re­gion where the peak po­ten­tial ex­ceeds the par­ti­cle’s ex­pec­ta­tion en­ergy by a fac­tor three.

Fig­ure 7.20: An tun­nel­ing par­ti­cle.

 

Move your mouse over the bot­tom fig­ure to see the an­i­ma­tion. Javascript must be en­abled on your browser. Give it a few sec­onds for the an­i­ma­tion to load, es­pe­cially on a phone line.
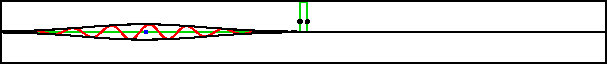
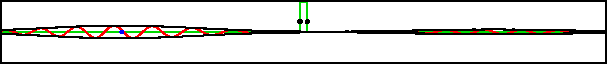
Im­proved res­o­lu­tion ver­sion (654 kB)

Of course, the en­ergy val­ues have some un­cer­tainty, but it is small. The rea­son the par­ti­cle can pass through is not be­cause it has a chance of hav­ing three times its nom­i­nal en­ergy. It ab­solutely does not; the sim­u­la­tion set the prob­a­bil­ity of hav­ing more than twice the nom­i­nal en­ergy to zero ex­actly. The par­ti­cle has a chance of pass­ing through be­cause its mo­tion is gov­erned by the Schrö­din­ger equa­tion, in­stead of the equa­tions of clas­si­cal physics.

And if that is not con­vinc­ing enough, con­sider the case of a delta func­tion bar­rier in fig­ure 7.21; the limit of an in­fi­nitely high, in­fi­nitely nar­row bar­rier. Be­ing in­fi­nitely high, clas­si­cally noth­ing can get past it. But since it is also in­fi­nitely nar­row, a quan­tum par­ti­cle will hardly no­tice a weak-enough delta func­tion bar­rier. In fig­ure 7.21, the strength of the delta func­tion was cho­sen just big enough to split the wave func­tion into equal re­flected and trans­mit­ted parts. If you look for the par­ti­cle af­ter­wards, you have a 50/50 chance of find­ing it at ei­ther side of this im­pen­e­tra­ble bar­rier.

Fig­ure 7.21: Pen­e­tra­tion of an in­fi­nitely high po­ten­tial en­ergy bar­rier.

 

Move your mouse over the bot­tom fig­ure to see the an­i­ma­tion. Javascript must be en­abled on your browser. Give it a few sec­onds for the an­i­ma­tion to load, es­pe­cially on a phone line.
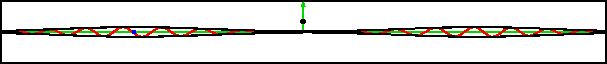
Im­proved res­o­lu­tion ver­sion (681 kB)

Cu­ri­ously enough, a delta func­tion well, (with the po­ten­tial go­ing down in­stead of up), re­flects the same amount as the bar­rier ver­sion.

Tun­nel­ing has con­se­quences for the math­e­mat­ics of bound en­ergy states. Clas­si­cally, you can con­fine a par­ti­cle by stick­ing it in be­tween, say two delta func­tion po­ten­tials, or be­tween two other po­ten­tials that have a max­i­mum po­ten­tial en­ergy $V$ that ex­ceeds the par­ti­cle's en­ergy $E$. But such a par­ti­cle trap does not work in quan­tum me­chan­ics, be­cause given time, the par­ti­cle would tun­nel through a lo­cal po­ten­tial bar­rier. In quan­tum me­chan­ics, a par­ti­cle is bound only if its en­ergy is less than the po­ten­tial en­ergy at in­fi­nite dis­tance. Lo­cal po­ten­tial bar­ri­ers only work if they have in­fi­nite po­ten­tial en­ergy, and that over a larger range than a delta func­tion.

Note how­ever that in many cases, the prob­a­bil­ity of a par­ti­cle tun­nel­ing out is so in­fin­i­tes­i­mally small that it can be ig­nored. For ex­am­ple, since the elec­tron in a hy­dro­gen atom has a bind­ing en­ergy of 13.6 eV, a 110 or 220 V or­di­nary house­hold volt­age should in prin­ci­ple be enough for the elec­tron to tun­nel out of a hy­dro­gen atom. But don’t wait for it; it is likely to take much more than the to­tal life time of the uni­verse. You would have to achieve such a volt­age drop within an atom-scale dis­tance to get some ac­tion.

One ma­jor prac­ti­cal ap­pli­ca­tion of tun­nel­ing is the scan­ning tun­nel­ing mi­cro­scope. Tun­nel­ing can also ex­plain al­pha de­cay of nu­clei, and it is a crit­i­cal part of much ad­vanced elec­tron­ics, in­clud­ing cur­rent leak­age prob­lems in VLSI de­vices.

Key Points

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

If the po­ten­tial varies non­triv­ially on quan­tum scales, wave pack­ets do not move like clas­si­cal par­ti­cles.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

A wave packet may split into sep­a­rate parts that move in dif­fer­ent ways.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

A wave packet may be re­flected by an ac­cel­er­at­ing force.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

A wave packet may tun­nel through re­gions that a clas­si­cal par­ti­cle could not en­ter.